For a long time economists have disagreed on how much hours of work and labour market participation rates respond to changes in wages. Labour economists estimate small labour supply elasticities from individual level data, while macroeconomists, who use business cycle fluctuations of wages and hours, argue that labour supply elasticities are considerably larger.1
Economists mostly have not, however, attempted to understand the pervasive and complex heterogeneity of labour supply, and the issues that this raises when we estimate aggregate effects.2 In this column we show there is substantial heterogeneity in women’s labour supply elasticities in cross section, and also over the business cycle. It is not possible to think about labour supply elasticity as a unique structural parameter.
What elasticity?
In this debate being precise about the type of wage variation that induces a labour supply response is key. Is it anticipated or unanticipated, temporary or permanent? Different types of variation generate different types of response, as argued by Blundell and MaCurdy (1999), among others. In a dynamic context, there are three different elasticity concepts that are relevant:
- The life-cycle Marshallian elasticity. This measures the change in hours worked and participation due to a change in the entire life-cycle wage profile.
- The life-cycle Hicksian elasticity. This measures the change due to the same change in the entire life-cycle wage profile, but after netting off the extra resources from the lifetime budget constraint. The life-cycle Hicksian elasticity is the right concept to discuss the impact of a funded change in the tax system that does not change the distribution of resources across individuals.
- The Frisch elasticity. This measures variation in labour supply due to anticipated change in the wage. The Frisch elasticity is the right concept to discuss the impact of business cycle fluctuations in wages.
An integrated approach to estimating elasticities
In a recent paper (Attanasio et al. 2018), we study women’s labour supply in the US using an integrated approach to estimate within-period and intertemporal preference parameters, and to map those parameters into the different concepts of elasticity. To do so, we use a life-cycle model in which unitary households make choices about consumption and women’s labour supply, given exogenous processes for male earnings and female wages.
Our specification of utility is flexible, and allows for different degrees of substitutability between consumption and leisure, intertemporal substitutability, and a rich role for demographic and other variables. Importantly, we follow recent contributions that have stressed the distinction between changes in labour supply at the extensive (participation) and the intensive (hours) margins to understand the aggregate response of labour supply (Chetty et al. 2011). We use data from Consumer Expenditure Survey (CEX) for the years 1980-2012.
Identification
We separate our estimation into three steps and specify what assumptions are needed at each step:
- We first identify within-period preferences over consumption and leisure at the intensive margin by estimating the MRS condition. We use group-level variability that is driven by group or aggregate shocks such as policy reforms. These parameters determinestatic Marshallian and Hicksian labour supply elasticities at the intensive margin. This is important because these elasticities can be a good approximation of the life-cycle Marshallian and Hicksian elasticities of interest.
- Next, we identify the inter-temporal preferences by estimating the Euler equation for consumption. We take into account the presence of unobserved taste shocks and use synthetic cohort data.
- Finally, we estimate the full life-cycle model and explicitly aggregate individual behaviour, as in Erosa et al. (2016).
Heterogeneity and aggregation
From a macroeconomic point of view, it is very important how individual responses translate into changes in aggregate hours of work. This is not trivial.
At the intensive margins, the simple specifications of preferences that fit the data give rise to non-linear expressions for the elasticities that do not aggregate in a simple fashion.
At the extensive margin it is even more complicated. The presence of non-convexities (such as fixed costs to go to work) induces clustering around kinks and corners of the budget constraint. The aggregate response therefore depends on the distribution of reservation wages, as noted by Chang and Kim (2006), and so the aggregate response will depend on the macroeconomic conditions of the economy. The response is likely to be time-varying and cyclical. Responses are likely to be higher after a sequence of shocks with the same size than after a period of relative calm.
These aggregation issues are relevant for both margins and undermine the very concept of a single aggregate labour supply elasticity.
Our findings
Our estimate of the curvature on hours worked in utility is much greater than the curvature on consumption: individuals are less willing to substitute hours of work over time than they are willing to substitute consumption. Further, we reject the restrictions on preferences required for balanced growth. Our estimates for the elasticities, at the median, are not too different from some of the estimates in the literature. In particular, on the intensive margin, we obtain a static Marshallian median elasticity of 0.18, with the corresponding static Hicksian elasticity considerably larger at 0.54, indicating a sizeable income effect.
For the same median household, the Frisch elasticity for hours is 0.87. However, we document considerable variation in the size of the estimated elasticities in the cross section. The Marshallian has an inter-decile range of -0.14 to 0.79, the Hicksian of 0.38 to 1.16 and the Frisch of 0.8 to 1.92. Figure 1 shows how this heterogeneity in elasticities relates to hours worked and the percentile of the wage distribution: elasticities are highest for those working the shortest hours and with the lowest wages. The heterogeneity we observe means it is not sensible to talk about a single elasticity measuring how labour supply in the economy responds to wage changes.
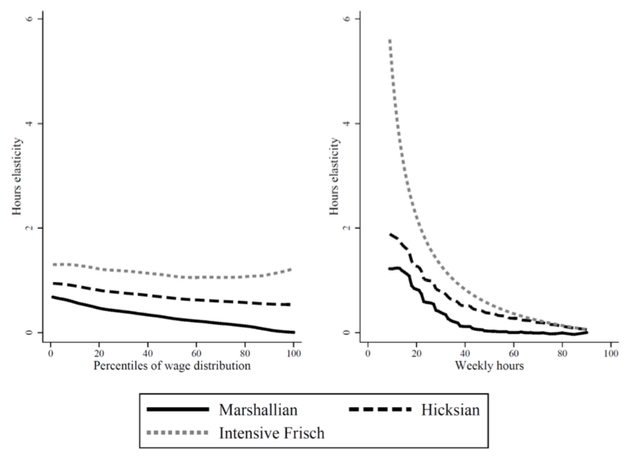
Source: Attanasio et al. (2018).
We use the entire model to study aggregate elasticities. We find an important role for the extensive margin in generating aggregate movements in labour supply, and further that aggregate movements depend on the heterogeneity in the population in terms of age, the number of children, and wealth.
Frisch elasticities are largest for the young and those with least wealth: the Frisch elasticity of aggregate hours goes from 1.93 in the age group 25 to 29 to 1.49 in the age group 50 to 55 and from 3.53 in the bottom quartile of the household’s wealth distribution to 0.81 in the top quartile. Over the business cycle, it varies from 1.53 in normal times to 1.61 in the first quarter of a recession to 1.71 after four quarters. Life-cycle elasticities are greater than the static because not all income is spent on nondurable consumption in the period it is earned.
Conclusion
The most important conclusion from our analysis is that the macro elasticity is not a structural parameter, it is simply the result of highly nonlinear aggregation which depends on demographic structure as well as the distribution of wealth and the particular point in the business cycle. This implies, for instance, that to understand the consequences of income tax changes, we need to be explicit about whose tax is changing: for some, such as women who are working few hours or on low wages, responses to changes in wages are substantial.
References
Attanasio, O, P Levell, H Low, and V Sanchez-Marcos (2018), “Aggregating Elasticities: Intensive and Extensive Margins of Women's Labour Supply”, Econometrica, forthcoming.
Blundell, R and T MaCurdy (1999), "Labor supply: A review of alternative approaches", Chapter 27 in O Ashenfelter and D Card (eds.), Handbook of Labor Economics, Volume 3, Elsevier.
Chang Y, and S Kim (2006), ‘’From individual to aggregate labor supply: a quantitative analysis based on a heterogenous agent macroeconomy”, International Economic Review 47: 1-27.
Chetty, R, A Guren, D Manoli, and A Weber (2011), “Are Micro and Macro Labor Supply Elasticities Consistent? A Review of Evidence on the Intensive and Extensive Margins”, American Economic Review 101: 471–475
Chetty, R (2012), “Bounds on Elasticities with Optimization Frictions: A Synthesis of Micro and Macro Evidence on Labor Supply", Econometrica 80(3): 969-1018.
Erosa, A, L Fuster, and G Kambourov (2016), “Towards a Micro-founded Theory of Aggregate Labor Supply", Review of Economic Studies 83: 1001-1039.
Keane, M P and R Rogerson (2012), “Micro and Macro Labor Supply Elasticities: A Reassessment of Conventional Wisdom”, Journal of Economic Literature 50: 464–476.
Keane, M P and N Wasi (2016), “Labor Supply: the Roles of Human Capital and the Extensive Margin", The Economic Journal 126: 578-617.
Martìnez, I, M Siegenthaler, and E Saez (2018), “Intertemporal labor supply substitution? Evidence from the Swiss Income Tax Holiday”, NBER working paper 24634.
Endnotes
[1] Keane and Rogerson (2012) and Chetty et al. (2011), among others, survey some of the issues and reasons for different estimates. Most recently, Martinez et al. (2018) estimate that the Frisch elasticity is essentially zero in Switzerland, which is very different to our recent results for the US.
[2] A notable exception, Keane and Wasi (2016) show male labour-supply responses vary substantially with age, education and the tax structure.
[3] Chetty et al. (2011).
This article was first published on VoxEU.












